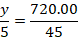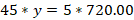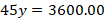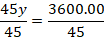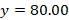One gallon of cleaning solution requires 10 oz of ammonia. If the maintenance department needs 51 gallons of solution to clean all of the floors, how much ammonia is needed?
A. 510 gallons
B. 51 gallons
C. 510 oz
D. 51 oz
We use given information to find how much ammonia is need to make the specified solution.
We are told, one gallon of cleaning solution requires 10 oz of ammonia. Expressing this mathematically yields two options:
\(\frac{10\ oz\ of\ ammonia}{1\ gallon\ of\ solution}\) or \(\frac{1\ gallon\ of\ solution}{10\ oz\ of\ ammonia}\)
Now we find how much ammonia is needed using option two.
\(51\ gallon\ of\ solution\ *\frac{10\ oz\ of\ ammonia}{1\ gallon\ of\ solution}\ =\ 510\ oz\ of\ ammonia\)
From the above equation, gallon of solution will cancel, and oz of ammonia is left.
Therefore, the solution will require 510 oz of ammonia.
Therefore, the Correct Answer is C.
More Questions on TEAS 7 Math
-
Q #1: When the marks of a science test are graphed, the distribution of markss is symmetric with the majority of weights centered around a single peak. Which of the following describes the shape of this distribution?
A. Uniform
B. Skewed right
C. Bell-shaped
D. Bimodal
Answer Explanation
In a bell-shaped curve, the data distribution is symmetric around a single peak. The centering of data around a single peak means the mean, mode and median of the test are all equal to each other.
-
Q #2: How many yards are in 27 feet?
A. 3 yards
B. 18 yards
C. 6 yards
D. 9 yards
Answer Explanation
Here we convert between feet and yards. We know 1 yard =3 feet. Then, 27 feet to yards will be:
Thus, 9 yards is equal to 27 ft.
-
Q #3: A large pizza has a diameter of 12 inches. Which of the following is the area of the pizza in terms of pi ()?
A. 24 πi n2
B. 48 π in2
C. 18π in2
D. 36 π in2
Answer Explanation
Before finding the area of the pizza, we need to find the radius of the pizza.
Find radius of the pizza in terms of diameter
Next, find area of the pizza using the radius of 6 inches
Substituting r=6 in the equation of the area of a circle
Thus, the pizza has an area of 36 π in2.
