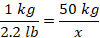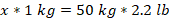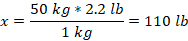The American with Disabilities Act (ADA) requires that the slope of a wheelchair ramp be no greater than 1:12. Which of the following is the minimum length of a ramp needed to provide access to a door that is 2.5 feet above the sidewalk?
A. 25 feet
B. 145 feet
C. 32.5 feet
D. 30 feet
The slope represent the ratio between the vertical height to the horizontal length. Let x be the minimum length of the ramp, we can set a proportion with height on the numerator and length on denominator. Then,
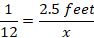
Cross-multiply to find the value of x
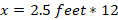
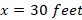
Thus, the minimum length of the ramp needed is 30 feet to access to a door that is 2.5 feet above the sidewalk.
Therefore, the Correct Answer is D.
More Questions on TEAS 7 Math
-
Q #1: Which of the following is the equivalence in pounds for 50 kg? (2.2 lb=1 kg)
A. 52.2 lb
B. 22.7 lb
C. 110 lb
D. 220 lb
Answer Explanation
We are asked to convert kg to pounds using the given relation. Letting x to represent the lb we are looking for.
Nest, we set the proportion with kg in the numerator and lb on the denominator as follows.
We find the value of x by cross-multiplication
The value of x is 110 lb.
-
Q #2: Which of the following is the mean of the test scores listed below? 100, 78, 47, 84, 93, 78
A. 80
B. 78
C. 96
D. 87
Answer Explanation
The mean of a data set is the total scores divided by the number of tests.
Total test scores =100+78+47+84+93+78=480
Number of tests =6
Mean test score =480/6=80
The mean test score is 80.
-
Q #3: Which of the following is an appropriate unit of measure to express the length of a dollar bill?
A. Decameters
B. Kilometers
C. Centimeter
D. Meters
Answer Explanation
Centimeter is the appropriate unit to measure the length of a dollar bill.
