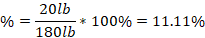Which of the following is the decimal form of 87.5%?
A. 8,750
B. 875
C. 8.75
D. 0.875
Percentages are fractions with denominator equal to 100. The given percent is divided by 100. To convert it to a decimal form, we move the decimal point two points to the left from the current position. Thus
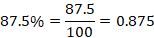
The decimal form of 87.5% is 0.875.
Therefore, the Correct Answer is D.
More Questions on TEAS 7 Math
-
Q #1: Which of the following is the independent variable in the equation below? F(t) = 4t + 9
A. f
B. 9
C. t
D. 4
Answer Explanation
An independent variable is a variable that is manipulated or changed in the experiment. From the given equation, t is an independent variable, which when changed changes the values of the f(t).
-
Q #2: A person weighed themselves at 180 lb. Three months later they weighed themselves at 160 lb. Which of the following is the percent of weight the person lost over 3 months? (Round to the nearest percent.)
A. 20%
B. 13%
C. 11%
D. 9%
Answer Explanation
The question requires us to find the percentage change in weight of a person.
First, we need to find the change in weight over the 3 months
Change in weight= 180-160=20 lb
Percent change in weight is change of original weight *100. Thus
The percent change in weight is 11% to the nearest whole number.
-
Q #3: Simplify the expression above. Which of the following is correct?
A. 6
B. 8
C. 1
D. 12
Answer Explanation
We follow the order of operations to solve the given expression.
First, we start with the numerator and solve it as follows
[2(3+5*3)]
We start with multiplication in inner brackets, 5*3=15. The expression becomes
[2(3+15)]
Then, we conduct the addition of 3+15=18. Then, the expression yields
[2(18)]=2*18=36
Now, we solve for denominator, which is 12/2=6.
Thus, the expression is reduced into
The expression reduces into 6.
